TRIGONOMETRY is the branch of maths to do with sin, cos and tan (find them on your calculator).
Literally it means “measuring triangles” – so you can use these three functions to find unknown lengths and angles.
Sin is short for “sine” – which is why it should be pronounced “sign” not “sinn”. Cos and tan are short for cosine and tangent – a nod to tan’s origins as the length of a tangent from a circle to the x-axis.
At GCSE we first introduce sin, cos and tan using the Mnemonic SOHCAHTOA in the context of right-angles triangles, and then develop the sine rule and cosine rule to deal with non-right-angled triangles.
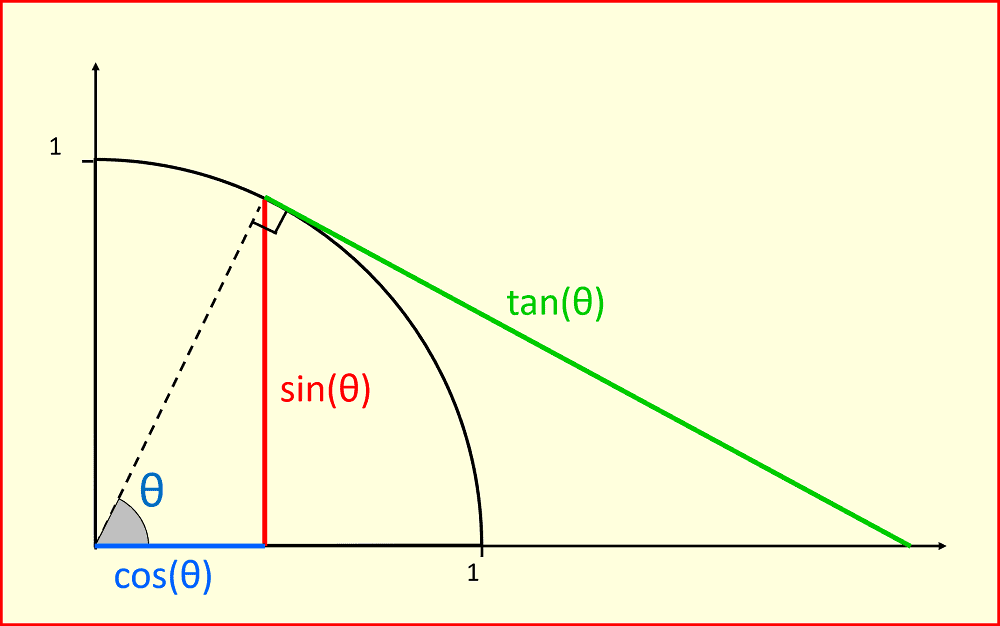
AT A-LEVEL: trigonometry gets a makeover! There are new better definitions of sin, cos and tan (see image), and three new friends sec, cosec and cot join the party. There are plenty of links between the six of them too. There are Compound Angle formulae, Pythagorean identities, double-angle identities and more.
WHAT’S THE POINT? As well as helping us to find distances and angles in triangles (and other polygons too), trigonometry is widely used to model objects moving in circles, like machine parts and fairground rides. The trig functions also have important links to complex numbers and e=2.71828… through the Further Maths A-level formula $e^{i\theta}=cos(\theta)+isin(\theta)$. Wow!